CAT Questions | CAT LR DI

CAT DI LR section has become increasingly tough beginning from 2015. However, Understanding the basics of Bar graphs, Pie Charts, Multiple graphs, Line Graphs etc forms an integral part of solving tougher CAT level DI LR questions for the CAT Exam. This question is from Logical Reasoning for CAT - Patterns.

Logical Reasoning for CAT: Patterns

Imperialist Gold Diggers

A group of six imperialists Alpha, Omega, Beta, Theta, Phi and Delta carve out a circle in Antartica and break it up into six sectors. There are 48 Gold mines spread across this circle that covers an area of 3600 sq kms.

Beta and Phi got the same area, while Theta and Delta got the same area. Area of Beta, Delta and Omega form an Arithmetic Progression with Delta being the middle term. Areas of Omega, Beta and Alpha form a Geometrics Progression with Beta being the middle term. The number of goldmines of Delta, Omega, Beta, Alpha and Theta form an Arithmetic Progression in that order. As many Countries have got more goldmines than Phi as have got fewer goldmines than Phi.

One has to cross at least two other Countries to go from Alpha to Delta; there is only one Country to cross between Phi and Omega; and Alpha and Beta are neighboring Countries. The Country that has got the maximum number of goldmines has an area of 450 sq kms, the Country that has got the maximum area has got 6 gold mines. Omega has lesser area than his neighbor Beta and Phi has got more goldmines than his neighbor Theta.

Question 5: How many more sq kms has Alpha got than has Beta?

Video Explanation

Explanatory Answer

Method of solving this Question on Logical Reasoning for CAT

General Solution

As ever, let us make some sense of the constraints and then draw a table or graph.

Areas - Beta = Phi = X, Theta = Delta = Y.

Area of Beta, Delta and Omega form an Arithmetic Progression with Delta being the middle term.

X, Y and Omega form an AP. Or, Omega = 2Y – X

Areas of Omega, Beta and Alpha form a Geometrics Progression with Beta being the middle term. Or, 2Y – X, X and Alpha form a GP.

Alpha = \\frac{X^2}{2Y - X}\\)

Now, let us move to the goldmines:

As many Countries have got more goldmines than Phi as have got fewer goldmines than Phi. There are totally 6 Countries, so Phi cannot be the lone middle term. So, Phi has to be joint third. So, we should have number of goldmines as P < Q < R=R < S < T.

Now, we know that the number of goldmines of Delta, Omega, Beta, Alpha and Theta form an Arithmetic Progression in that order. So, the number of goldmines of Beta and Phi should be same, say R. Then we have R – 2d, R- d, R, R, R + d, R + 2d. The sum of all these is 6R. Or, R = 8.

So, Phi and Beta have 8 goldmines each.
Now, let us create a table and work from there



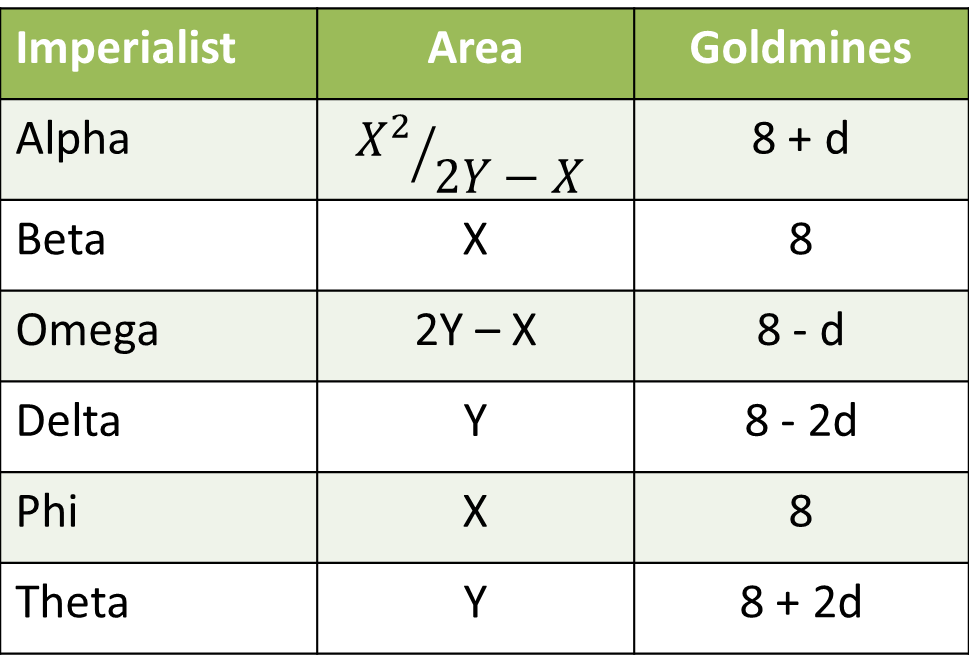
Omega has lesser area than his neighbor Beta . Or, 2Y – X < X. Or, Y < X.
and Phi has got more goldmines than his neighbor Theta. Or, d < 0.
8 + d and 8 + 2d should be less than 8, whereas 8 – d and 8 – 2d should be higher than 8. The Country that has got the maximum number of goldmines has an area of 450 sq kms. Or, Delta has an aread of 450 sq kms. Y = 450 sq kms.

The Country that has got the maximum area has got 6 gold mines. Alpha or Theta should have 6 gold mines.

Now, we know Y < X. So, Theta does not have the maximum area. So, alpha should have the maximum area. SO, 8 + d=6. Or, d=-2. We have cracked the goldmine part completely. Let us include this in our table.


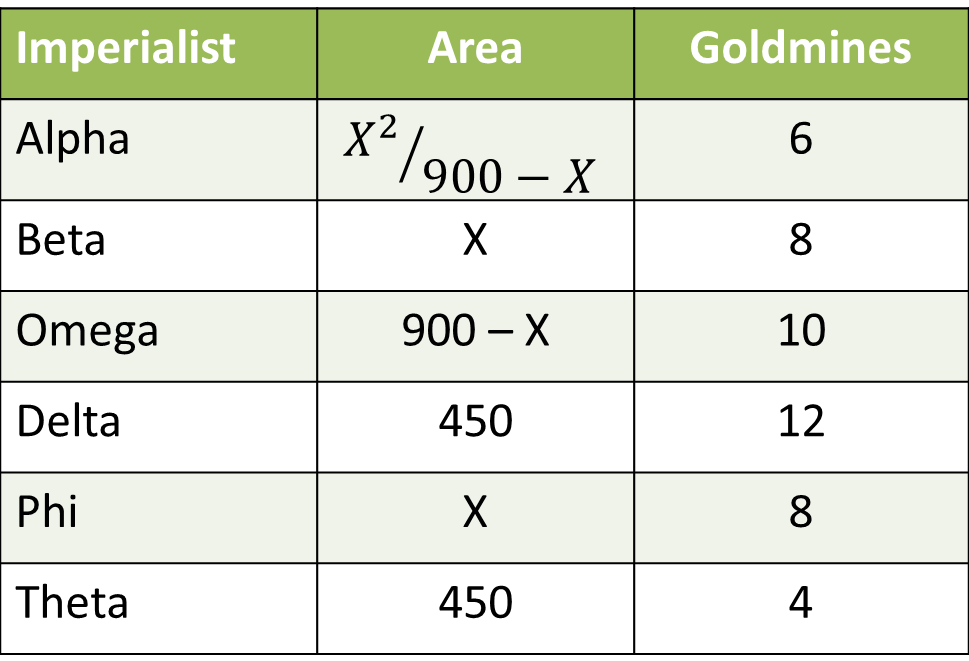
Total area is 3600 sq kms. Or, \\frac{X^2}{900 - X}\\) + X + 900 +X + 450+X + 450 = 3600.

Or \\frac{X^2}{900 - X}\\) + X = 1800
X² +900X -X² =1800(900-X)

Solving, we get X = 600, so 900 - X = 300.
The table now looks like:


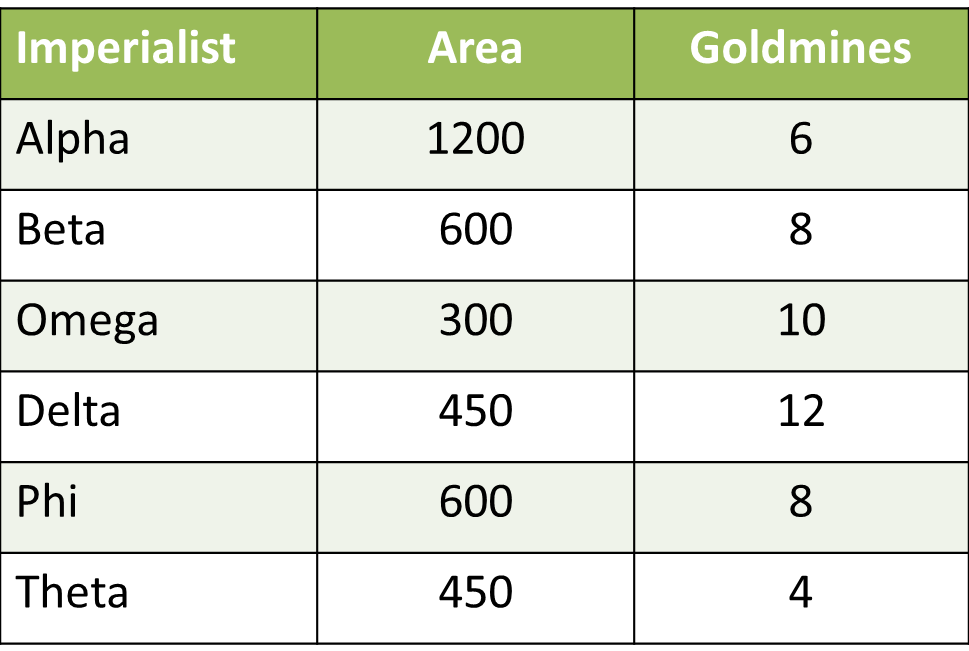
Now, let us move on to the positions on the grid. One has to cross at least two other Countries to go from Alpha to Delta – Alpha and Delta are diametrically opposite to each other ( athough the sectors might have different areas) Alpha and Beta are neighboring Countries. Omega has lesser area than his neighbor Beta. Or, Beta sits between Alpha and Omega. So, we have Alpha, Beta, Omega in a sequence.

Alpha and Delta are diametrically across. So, we should have Alpha, Beta, Omega and Delta in a sequence.

There is only one Country to cross between Phi and Omega. One Country removed from Omega in one direction is Alpha. In the other direction it should be Phi. So, we should have Alpha, beta, Omega, Delta, Phi in a sequence.

Phi has got more goldmines than his neighbor Theta.
Or, the sequence is Alpha, Beta, Omega, Delta, Phi, Theta.


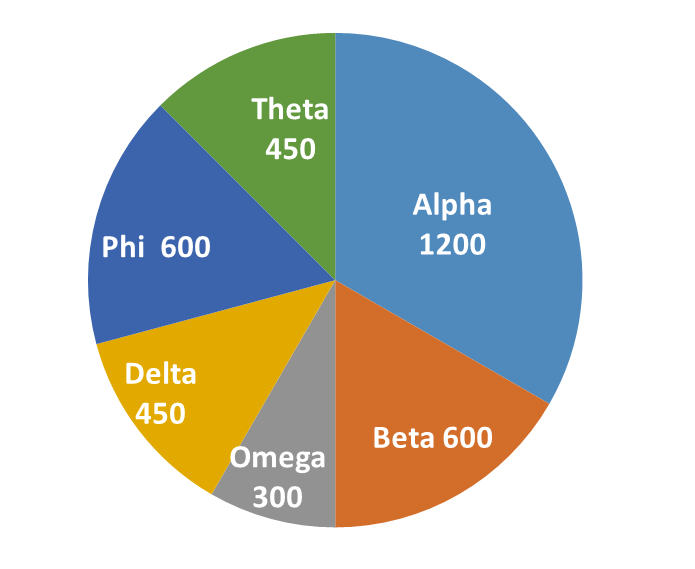
From the chart, the answer is 600 sq km.

The question is "How many more sq kms has Alpha got than has Beta?"

Hence, the answer is "600 sq km".